Planar & Round Jets: Analysis of Particle Motions & Statistics in High Reynolds Number flows

 Coflowing Round Jet

Tracers in a round turbulent jet (coflow = ½)

Velocity Excess in Coflowing Round Jet vs. Streamwise Position

Uexcess = (Ucenterline – Ucoflow)/Ucoflow

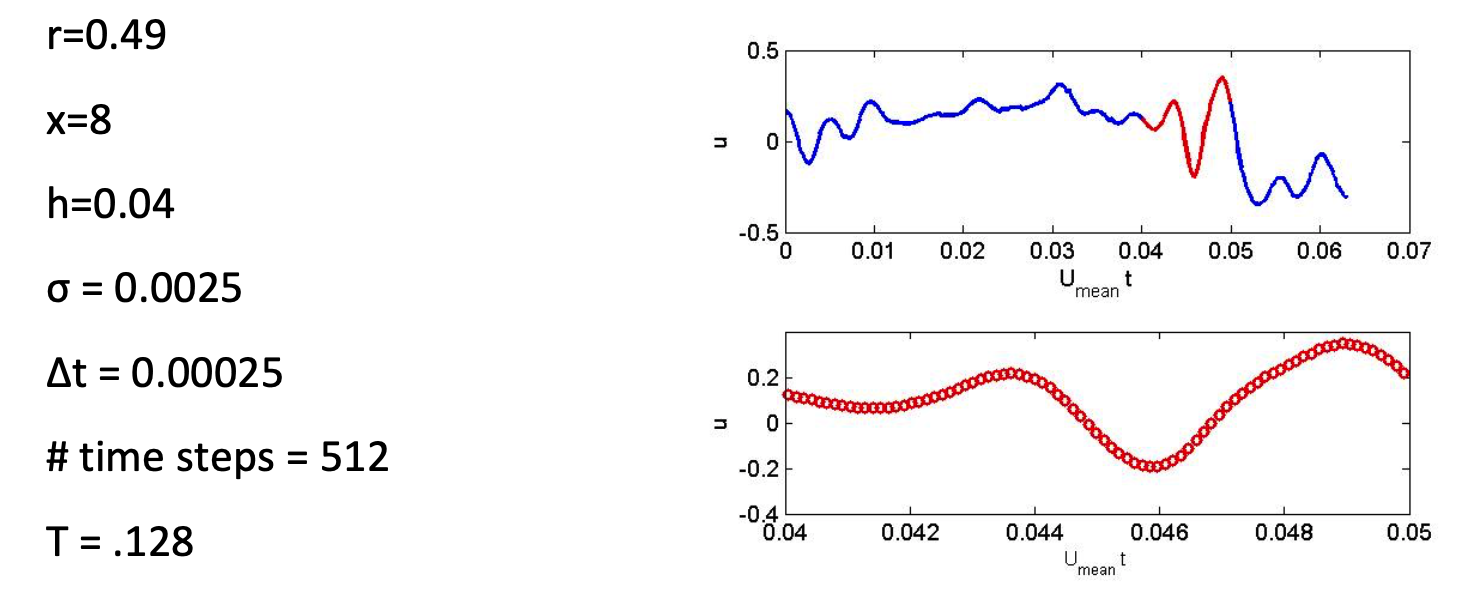
Numerical Parameters

• h – tube length   

• Δt  - time step

• G  - circulation   

• σ  - smoothing parameter in Biot-Savart Law 

• d - criterion for loop removal 

Reynolds Number of a Turbulent Blob

• h=0.005

• Velocity data acquired on spanwise lines: typically, 625 lines, 2500

Statistics include:

• velocity variances

• longitudinal and transverse

2pt velocity correlation

functions → microscales

• structure functions

Sp(r)=|u(x+r)-u(x)|p

• 1D energy spectra points/line

Two point longitudinal, f(r), and transverse, g(r), correlation functions – note the strong dependence on σ.

Symbols show consistency with the isotropy condition:

1D Energy Spectra in Kolmogorov Units

Statistics of Particle Accelerations

Tracers determined from fine-grained calculations at small Δt:

• vortical field evolves with tracers.

Acceleration data acquired from sampling along paths:

• mainly determined from derivative of piecewise parabolic fits to velocity data

Loop removal appears to create outlier events that add to (or cause)

• Δt dependence of moments. However, PDF’s are relatively unaffected.

Turbulent blob

Coflowing round jet

Variance and flatness statistics:

 Δt = 0.005, σ = 0.005, 

varx = 3.72,  vary = 4.06,  varz = 3.69

fx = 28.4,  fy = 24.9,  fz = 21.9

 Re=546623   Rλ=442  ε= 0.016

a0 = varx  Re1/2  ε3/2     = 2.49

End on view of tracers in coflowing  round jet.

Note the intermittency

PDFs of fluid particle accelerations: coflowing round jet

Acceleration auto-correlation for round jet

Conclusions:

• Grid free vortex filament simulations including loop removal as a de facto “subgrid” model provide a useful avenue for simulating complex high Reynolds number flows.

• A small tube length is necessary for accurate Reynolds stress predictions.

• The general consistency with Kolmogorov inertial range scaling allows for the determination of local Reynolds number Rλ.

• For a given set of numerical parameters, Rλ can be expected to increase as σ decreases. In fact, Kolmogorov length scale ≈ 1/5 σ.

• PDFs of fluid accelerations in the blob and round jet flows are consistent with physical experiments in other contexts.

• Outlier events caused by loop removal complicate the determination of moments.